Knots representing numbers: The mathematics of the Incas

A favorite mantra among mathematicians, originally attributed to Galileo Galilei, says that the universe is written in a mathematical language. But while math may be our common language, the way we represent its concepts can often be quite different. Faced with the basic need to count, for example, societies developed numbers, but in every place where counting arose, methods of representing quantities often varied drastically: Babylonians used the sexadecimal system and wrote numbers using notches on clay tablets; the Greeks used letters; and the Incas, for their part, employed quipu, or knotted strings. Inca quipu (sometimes spelled khipu) have found renewed interest in the Spanish-speaking world with the recent publication of El espía del Inca (or The Inca’s Spy), a monumental novel by Peruvian author Rafael Dumett, the protagonist of which is a quipus weaver with extraordinary skills in arithmetic.

Quipu were invented to convey information, sometimes in code, between anywhere in the Inca Empire – an area that encompassed a large portion of South America and was as vast and varied as the Roman Empire – to the capital of Cuzco, in what is now Peru. The word quipu comes from Quechua and means “knot.” And this is precisely what quipu are: knots used to represent mathematical concepts, i.e., numbers. Interestingly, centuries later, modern mathematics would again turn to the study and classification of knots, knot theory, and the topology of knots.

The oldest known quipu date back to 2,500 B.C. and were used for millennia, until the process of Spanish colonization led to their obsolescence. Spanish conquistadors considered the objects idolatrous and dangerous, and would destroy the knotted ropes by burning them. Quipu were usually made of cotton, or from the wool of llamas or alpacas. They are created using one main top rope with no knots, from which other knotted strings of different colors, shapes and sizes were tied in rows. The colors of the strings represent different categories – for example, brown corresponds to government; crimson to the Inca sovereign, ruler of the empire; and red to war – while the knots indicate quantities, including the number zero, which is represented by the absence of knots.

Each string contains a group of knots that together represent a number. In their book Mathematics of the Incas: Code of the Quipu, authors Marcia Ascher and Robert Ascher – a mathematician and an anthropologist, respectively – analyzed hundreds of quipu examples and determined that the Inca counting system uses three fundamental types of knots: simple, one-turn knots (s); long knots with one or more additional turns (L); and figure-eight knots (E). The letters that Ascher and Ascher used to represent quipu numbers is known as the “Ascher system.”

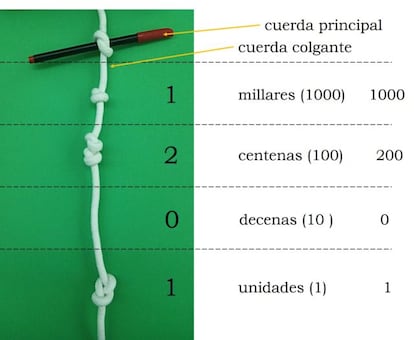
Like our Indo-Arabic numeral system, Inca quipu use a base-ten decimal system. The knots have different positions on the string, which represent different numbers and powers of ten – ones, and then tens, hundreds, thousands, etc. The digits for units of one are represented by long knots – for example, 4 is represented using one knot with four turns. Due to the way the knots are tied, the number 1 cannot be shown in this way, so it is represented by a figure-eight knot.

Digits in positions of 10 and higher powers are represented by groups of simple knots. For example, 40 is represented using four simple knots tied in a row in the ten position. Zero is represented by the absence of a knot where one would otherwise be. In the Ascher system, the zero knot is denoted with an X. Thus, 804 is described as 8s, X, 4L.

In Inca society, those responsible for making quipu were called quipucamayoc, and they studied their trade in the yachay wasi, or “house of teaching.” The quipucamayoc knew how to add, subtract, multiply and divide, but they were not merely accountants; they belonged to the nobility, were required to be over fifty years old, and constituted a closed caste in the Inca hierarchy.

The quipucamayoc did not use quipu to perform mathematical operations – the knots served only to record and communicate numbers. For that task, they used a kind of abacus called a yupana (“count” in Quechua), which used kernels of corn to conduct arithmetic calculations. How the yupana worked is still not entirely clear, but it is believed to be similar to other abacuses developed in Europe and Asia.

Like much of Inca culture, knowledge of the yupana eventually vanished with the arrival of the Spanish and the subsequent process of colonization; the Incas never developed writing, so there are no written records of the tool’s use. But a remnant of the art of Inca mathematics has been preserved in the civilization’s knotted ropes. One cannot help but wonder how this technique, and the mathematical arts of the Incas, would have evolved without the intervention of the Spanish conquest.

Manuel de León is a research professor at the Institute of Mathematical Sciences (ICMAT), an institute of the Consejo Superior de Investigaciones Científicas (Spanish National Research Council), and a member of the Real Academia de Ciencias Exactas, Físicas y Naturales (Royal Academy of Exact, Physical and Natural Sciences).

Sign up for our weekly newsletter to get more English-language news coverage from EL PAÍS USA Edition